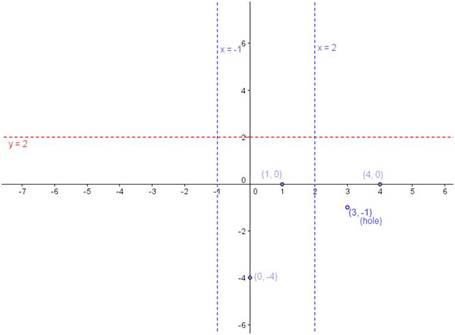
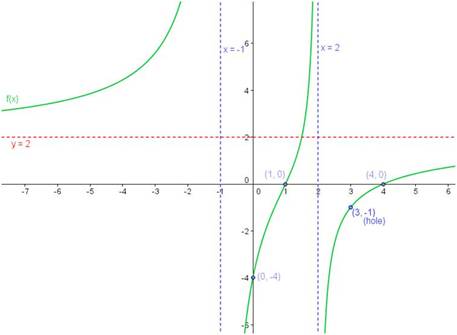
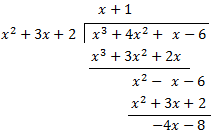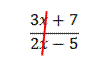Bay Area Tutoring

A mnemonic for remembering values on the unit circle

By Tutor GuyNo Comments

In an earlier post, I told you that you must memorize the values on the unit circle if you want to be great at trigonometry. So let’s say that you have taken my advice, but on a test you have a big brain cramp and you forget the values. Here’s a trick that will help you fill in the values in the first quadrant. The five angles in the first quadrant are 0°, 30°, 45°, 60°, and 90°. And the sines of these angles just happen to be:

$\underline{ \qquad \theta \qquad \sin \theta \qquad \qquad \qquad}$

$0 \textdegree \qquad \; \; 0 \qquad =\dfrac{\sqrt{0}}{2}$

$30 \textdegree \qquad \dfrac{1}{2} \qquad = \dfrac{\sqrt{1}}{2}$

$45 \textdegree \qquad \dfrac{\sqrt{2}}{2} \quad = \dfrac{\sqrt{2}}{2}$

$60 \textdegree \qquad \dfrac{\sqrt{3}}{2} \quad = \dfrac{\sqrt{3}}{2}$

$90 \textdegree \qquad 1 \qquad = \dfrac{\sqrt{4}}{2}$

Do you see the pattern in the last column? The sines increase from

$\dfrac{\sqrt{0}}{2}$ to $\dfrac{\sqrt{4}}{2}$

That’s an easy pattern to remember even during the worst brain cramp. Why does it work? It’s just a coincidence. But if you use this pattern, you can fill in the sine values in the first quadrant. Then the cosine values are the same in reverse order. Once you’ve completed the first quadrant, you can fill in the rest of the unit circle by using reference angles. Now you have a completed unit circle!

Sep 22

Using the reciprocal key on your calculator

By Tutor GuyNo Comments

Your graphing calculator has a reciprocal key on it, designated as $x^{-1}$ . It’s the easiest way to find the reciprocal of a number on your screen. Just press the key, and then Enter.

The reciprocal key is also a great way to do calculations of resistors in parallel or capacitors in series. For example, find the equivalent resistance of a circuit that has a 10 Ω and a 15 Ω resistor in parallel. Simply enter it this way into the calculator to find the answer is 6 Ω.

Sep 15

Factoring the difference of two squares

By Tutor GuyNo Comments

You will spend a lot of time in algebra (and courses beyond) factoring polynomials into linear and quadratic terms. There are some special polynomials that occur so frequently that you should recognize them on sight so that you know the method for factoring them. The most common is the binomial of the form $a^2-b^2$ . This is called the difference of two squares because both terms are perfect squares and you are subtracting (finding the difference between) the two terms.

Some examples of this binomial are $x^2-4$ , $9x^4-16y^2$ and $121y^2z^2-25w^4a^6b^{12}$ . Note that in each case, both terms are perfect squares.

It is very easy to factor these expressions—you can do it by inspection. The rule is simple and you must memorize it: $a^2-b^2=(a+b)(a-b)$ . Once you determine that an expression is the difference of two squares, you can write out its factors immediately. Let’s see how it works with the three examples given above:

$x^2-4=(x+2)(x-2)$

$9x^4-16y^2=(3x^2+4y)(3x^2-4y)$

$121y^2z^2-25w^4a^6b^{12}=(11yz+5w^2a^3b^6)(11yz-5w^2a^3b^6)$

It’s really that easy once you learn the format. Of course it doesn’t matter what order you write the two factors, so $x^2-4=(x-2)(x+2)$ is also correct.

One other important point: this factoring rule works in reverse, too! If you have to distribute (multiply out) two factors and you see they are in the form of $(a+b)(a-b)$ , you should recognize that this gives the difference of two squares: $a^2-b^2$ . You can do this immediately, without multiplying out every term. Learning this formula will save you lots of time.

Sep 01

Graphing rational functions—4. Drawing the graph

By Tutor GuyNo Comments

In previous posts, I described the steps you follow to analyze a rational function. If you follow these steps, you can be a rational function superstar too. In this post, I put all the pieces together to show how you use the information you’ve obtained to plot the graph. [For details on how to execute the various steps, please see other posts on this website.]

Example: Graph the following rational function.

$f(x)=\dfrac{2x^3-16x^2+38x-24}{x^3-4x^2+x+6}$

Solution: We break this into many steps.

1. Find the $y$ -intercept:

$f(0)=\dfrac{-24}{6}=-4$

2. Fully factor the function:

$f(x)=\dfrac{2x^3-16x^2+38x-24}{x^3-4x^2+x+6}=\dfrac{2(x-1)(x-3)(x-4)}{(x+1)(x-2)(x-3)}$

3. Find the vertical asymptotes, if any:

The vertical asymptotes are at $x=-1$ and $x=2$ .

4.Find the $x$ -intercept(s), if any:

$f(x)=0$ @ $x=1$ and $x=4$

5. Find the hole(s), if any:

There is a hole at the point $(3,-1)$

6. Find the horizontal or oblique asymptote, if any, or characterize the end behavior:

The horizontal asymptote is at $y=2$

7. Place all of this information on the graph:

8. Use all the information you have plotted to complete the graph of the function:

Aug 25

Graphing rational functions—3. Finding horizontal or oblique asymptotes

By Tutor GuyNo Comments

In a previous post, I listed the steps you need to follow to analyze and graph a rational function. In this post, I show you how to find the horizontal or oblique asymptotes, if they exist. If there are no horizontal or oblique asymptotes, then you can determine the end behavior of the function. [Check other posts on this website for other steps in analyzing and graphing a rational function.]

The horizontal and oblique asymptotes, if they exist, tell you the end behavior of the function. That is, they describe what the function is doing as $x$ goes to ±∞. In order to determine the end behavior, examine the degrees of the polynomials in the numerator and denominator. There are four possible cases:

Case 1: The degree of the denominator is greater than the degree of the numerator.

When the denominator has the higher degree, the denominator grows much faster than the numerator as $x$ gets very large. Like all fractions, as the denominator gets very large, the fraction gets very small and approaches zero. So the asymptote is $y=0$ .

Example 1: Find the end behavior for the following rational function:

$f(x)=\dfrac{x^2+3x+2}{x^3+4x^2+x-6}$

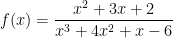
Solution: Because the degree of the numerator is 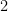$2$ and the degree of the denominator is $3$ , this function has a horizontal asymptote $y=0$ .

Case 2: The degree of the denominator is equal to the degree of the numerator.

When the degrees of the numerator and denominator are the same, the function approaches a finite non-zero number. This number is the ratio of the leading coefficients of the two polynomials and this is the value of the horizontal asymptote.

Example 2: Find the end behavior for the following rational function:

$f(x)=\dfrac{6x^2+7x-4}{5x^2-4x-6}$

Solution: Because the degree of the numerator and denominator are the same, this function has a horizontal asymptote. Divide the leading coefficients (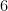 $6$ and $5$ ) and the horizontal asymptote is $y=6/5$ .

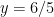Case 3: The degree of the numerator is exactly one higher than the degree of the denominator.

In this case, there is an oblique asymptote (called a slant asymptote in some textbooks). To find the asymptote, divide the denominator into the numerator using long division. The quotient is the oblique asymptote. (Ignore the remainder; it has no effect on the asymptote.)

Example 3: Find the end behavior for the following rational function:

$f(x)=\dfrac{x^3+4x^2+x-6}{x^2+3x+2}$

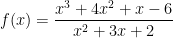
Here, the numerator (degree 3) is exactly one higher than the denominator (degree 2). To find the asymptote, perform the following long division:

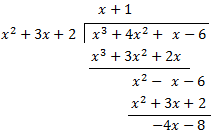
The quotient is the oblique asymptote:

$y=x+1$

Case 4: The degree of the numerator is more than one higher than the degree of the denominator.

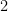In this case, there is no horizontal or oblique asymptote. Instead, the end behavior of the function is the same as the end behavior of a polynomial whose degree is the same as the difference between the degrees of the numerator and the denominator. For example, if the numerator is degree $5$ and the denominator is degree $2$ , the rational function will look like a cubic function as $x$ approaches ±∞.

Aug 18

Graphing rational functions—2. Finding holes and vertical asymptotes

By Tutor GuyNo Comments

In a previous post, I listed the steps you need to follow to analyze and graph a rational function. In this post, I show you how to find the hole(s) and vertical asymptote(s), if they exist. [Check other posts on this website for other steps in analyzing and graphing a rational function.]

In a rational function, holes and vertical asymptotes will only occur where the denominator is equal to zero. So fully factor the function, and look for the roots of the denominator. When a factor in the denominator cancels out with a factor in the numerator, the function will have a hole where the factor equals zero. If the factor in the denominator does not cancel out with a factor in the numerator, there will be a vertical asymptote where the factor equals zero.

Example: Find the holes and vertical asymptotes, if any, for the following rational function:

$f(x)=\dfrac{x^2+3x+2}{x^3+4x^2+x-6}$

First, fully factor the function:

$f(x)=\dfrac{x^2+3x+2}{x^3+4x^2+x-6}=\dfrac{(x+1)(x+2)}{(x-1)(x+2)(x+3)}=\dfrac{(x+1)}{(x-1)(x+3)}$

The roots of the denominator are $-3$ , $-2$ and $1$ . At $x=-2$ , there is a hole. To find the “value” of the hole, evaluate the reduced function:

$f(-2)=\dfrac{(-2+1)}{(-2-1)(-2+3)}=\dfrac{-1}{-3}=\dfrac{1}{3}$

This means that when you graph the function, there will be a hole at the point $(-2, 1/3)$ .

The vertical asymptotes will be $x=-3$ and $x=1$ .

Aug 11

Graphing rational functions—1. Finding x- and y- intercepts

By Tutor GuyNo Comments

In a previous post, I listed the steps you need to follow to analyze and graph a rational function. In this and subsequent posts, I will walk you through each of those steps. Usually the first step in plotting a rational function is finding the $x$ – and $y$ -intercepts. You have done this before for other functions and the process here is no different.

To find the $y$ -intercept, set $x=0$ and solve for $f(x)$ .
To find the $x$ -intercept(s), set $f(x)=0$ and solve for $x$ . Recall that a rational function is a fraction, and a fraction can only be equal to zero when its numerator is equal to zero (as long as the denominator is not also equal to zero!). So in practice, you find the $x$ -intercept(s) by setting the numerator equal to zero and solving for $x$ .

Example: Find the $x$ – and $y$ -intercepts, if any, for the following rational function:

$f(x) = \dfrac{x^3+4x^2+x-6}{x^2+3x+2}$

Finding the $y$ -intercept is very easy if the polynomials are written in standard form as above. $f(0)$ is simply the ratio of the constant terms: $\frac{-6}{2} = -3$ . If the rational function has already been factored, you need to plug zero in to each term and evaluate.

To find the $x$ -intercept (and all the other parts of the rational function), the first thing you need to do is fully factor the numerator and denominator.

$f(x)=\dfrac{x^3+4x^2+x-6}{x^2+3x+2}=\dfrac{(x-1)(x+2)(x+3)}{(x+1)(x+2)}$

From here, it is easy to see that the roots of the numerator are $-3$ , $-2$ and $1$ . When $x=-2$ , the denominator is also zero, so this is not an $x$ -intercept; it is a hole. The $x$ ‑intercepts are at $-3$ and $1$ .

Is it possible that a rational function does not have a $y$ -intercept? Yes, if the function is not defined for $x=0$ . In other words, if a rational function has either a hole or a vertical asymptote at $x=0$ , there is no $y$ -intercept.

[Check out my other posts in this section that describe how to perform the other steps when analyzing a rational function.]

Aug 04

Graphing rational functions—overview

By Tutor GuyNo Comments

Graphing rational functions can be scary for a lot of students because there are so many details to manage. But you can make the process simpler by breaking down all the requirements into small steps. In this post I list all of the steps you should follow to analyze a rational function. Note that you can do these steps in any order. In subsequent posts, I will walk you through each of the steps and show you how to execute them.

Let’s start with the definition of a rational function. A rational function is a function that consists of a fraction, where both the numerator and denominator are polynomials. Or in symbols, if g(x) and h(x) are polynomials, then

$f(x)=\dfrac{g(x)}{h(x)}$

is called a rational function.

To analyze and graph a rational function, you need to do all of the following steps:

Find the y-intercept (if it exists)
Find the x-intercept(s) (if they exist)
Determine the location of holes, if any
Find the vertical asymptotes, if any
Find the horizontal or oblique asymptotes, if any
Determine the end behavior (if no horizontal or oblique asymptotes)

[Note that this is a list for algebra 2 and precalc students. When you get to calculus, there will be additional steps to completely analyze the function.]

This probably feels like a long and complicated list, but for the most part, each step by itself is not very difficult. Just perform each step in turn and you can become an expert at rational functions. Check out my other posts in this section that describe how to perform each of these steps.

Jul 21

The Most Common Factoring Mistake

By Tutor GuyNo Comments

I am surprised (and a little disappointed) every year when one of my students tries to simplify a polynomial fraction by cancelling out terms that can’t be cancelled out. For example, when faced with

$\dfrac{3x+7}{2x-5}$

inevitably, a student will ask me, “Can I cancel out the x’s like this?”

Tears well up in my eyes as I explain that no, the x’s do not cancel. I explain patiently why the x’s do not cancel. And very often, the next time I work with that student, he or she will try to cancel out the x’s again. This is the most common factoring mistake I see students make, and it’s not limited to Algebra students. I’ve even seen Calculus student make this error. That usually makes me sob quite loudly.

If you would like to keep me from crying, then you need to learn how to simplify polynomial fractions. It’s quite simple once you understand that terms that are added do not cancel out. Only factors that are multiplied together can cancel. Let’s start by looking at a fraction with numbers and no variables.

$\dfrac{210}{462}$

Can this be simplified? Of course. Most students will divide the top and bottom by 2, then by 3, and then by 7, as follows:

$\dfrac{210}{462}=\dfrac{105}{231}=\dfrac{35}{77}=\dfrac{5}{11}$

This is correct. But why can you cancel out a 2 and a 3 and a 7? It’s because they are factors of the numerator and the denominator. Let’s do the same problem by completely factoring the top and bottom first:

$\dfrac{210}{462}=\dfrac{2\cdot 3 \cdot 5 \cdot 7}{2 \cdot 3 \cdot 7 \cdot 11}=\dfrac{5}{11}$