Archive

Apr 13

Integrals involving trig substitutions

By Tutor GuyNo Comments

 

When is it appropriate to solve an integral with a trig substitution? First of all, keep in mind that a trig substitution doesn’t always work. Even when it does work, you are often left with an integral that will require other techniques such as a u substitution or integration by parts. But if you are willing to put in a little effort (and you know your trig identities), trig substitutions allow you to find the antiderivatives of some rather complicated functions.

There are three conditions that you look for—each a radical term of a particular form in the integrand. Each condition is associated with a different substitution. After you make the substitution, you simplify the integrand and go from there.

Term

Substitution

Radical becomes…
\sqrt{a^2-x^2} \text{Let } x=a \sin \theta; \, dx=a \cos \theta \,d \theta a \cos \theta
\sqrt{a^2+x^2} \text{Let } x=a \tan \theta; \, dx=a \sec^2 \theta \,d \theta a \sec \theta
\sqrt{x^2-a^2} \text{Let } x=a \sec \theta; \, dx=a \sec \theta \tan \theta \,d \theta a \tan \theta

 

Before we look at some example integrals, let’s see why the first radical term above simplifies to a \cos \theta. It’s pretty straightforward if you know your trig identities:

\sqrt{a^2-x^2}=\sqrt{a^2-(a \sin \theta)^2}= \sqrt{a^2-a^2 \sin^2 \theta}= \sqrt{a^2(1- \sin^2 \theta)} =

 …….. \sqrt{a^2 \cos^2 \theta} = a \cos \theta

Example. Integrate the following:

\text{a.} \displaystyle \int \frac{dx}{\sqrt{1-x^2}} \qquad \text{b.} \int \frac{x^3}{8 \sqrt{4+x^2}}dx \qquad \text{c.} \int x \sqrt{x^2-4} \; dx

Solutions:

  1. (Does this integral look familiar?) Here, a=1, so use x= \sin \theta. Using the first line of the table above:
    …..
    \displaystyle \int \frac{dx}{\sqrt{1-x^2}}= \int \frac{\cos \theta \, d \theta}{\cos \theta}= \int d \theta = \theta +C
    …..
    But since x= \sin \theta, \theta=\sin^{-1} x.
    …..
    \therefore \displaystyle \int \frac{dx}{\sqrt{1-x^2}}=\sin^{-1} x+C
  2. Here, a=2 and we use line 2 from the table above (x=2 \tan \theta). Note that x^3=8 \tan^3 \theta. Upon substitution,
    …..
    \displaystyle \int \frac{x^3}{8 \sqrt{4+x^2}}\, dx= \int \frac{8 \tan^3 \theta}{8(2 \sec \theta)}2 \sec^2 \theta \, d \theta = \int \tan^3 \theta \sec \theta \, d \theta
    …..
    Hmm. This is going to take a little bit of extra work… Time to pull out some trig identities:
    …..
    \int \tan^3 \theta \sec \theta \, d \theta = \int \tan^2 \theta \tan \theta \sec \theta \, d \theta = \int (\sec^2 \theta -1) \tan \theta \sec \theta \, d \theta
    …..
    Now a u substitution, letting u= \sec \theta:
    …..
    \displaystyle \int (u^2-1) \, du= \frac{1}{3} u^3-u+C= \frac{1}{3} \sec^3 \theta - \sec \theta +C
    …..
    How do we get our answer back in terms of x? Draw a triangle that shows how x and \tan \theta are related, then use the Pythagorean theorem to find an expression for \sec \theta. In the triangle below,
    …..
    x=2 \tan \theta \therefore \sec \theta = \dfrac{\sqrt{4+x^2}}{2}
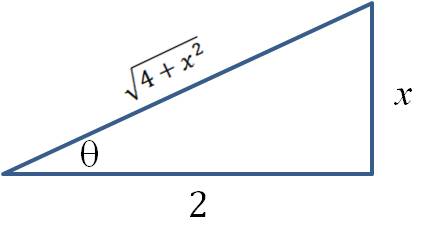    Substitute into the integral above to get:
    \displaystyle \int \frac{x^3}{8 \sqrt{4+x^2}} dx=\frac{1}{3} \sec^3 \theta - \sec \theta +C= \frac{(4+x^2)^{3/2}}{24}- \frac{(4+x^2)^{1/2}}{2}+C

    This can be simplified further by factoring:
    …..
    \displaystyle \int \frac{x^3}{8 \sqrt{4+x^2}}dx= \frac{(4+x^2)^{3/2}}{24}- \frac{(4+x^2)^{1/2}}{2}+C= \frac{1}{24}(x^2-8) \sqrt{4+x^2}
    …..
    Whew!

  3. This is a trick question. Even though it fits the condition given in the table (and you could integrate with a trig substitution if you wanted), it’s easier to do this one with a u substitution: u=x^2-4 and du=2x \; dx:
    …..
    \displaystyle \int x \sqrt{x^2-4} \, dx= \frac{1}{2} \int \sqrt{u} \, du= \frac{1}{3}u^{3/2}+C= \frac{1}{3}(x^2-4)^{3/2}+C

The lesson here is to look for u substitutions before you look for trig substitutions.

Apr 06

Using f”(x) to interpret f’(x)

By Tutor GuyNo Comments

 

You know that the second derivative of a function is used to characterize the concavity of the function. But did you know that the second derivative also gives you information about the first derivative? Well, of course it does, because the second derivative is the first derivative of the first derivative. To put it simply, when the second derivative is positive, that means the first derivative is increasing. When the second derivative is negative, the first derivative is decreasing.

But wait, I thought when the second derivative is positive, that means the original function is concave up! Well, yes, that’s true too. So that means wherever a function is concave up, its first derivative is increasing. And wherever a function is concave down, the first derivative is decreasing.

This is another way you can analyze the behavior of a function.

Mar 23

Using multiplicity of factors to characterize graphs of rational functions

By Tutor GuyNo Comments

 

Rational functions can be scary because there are so many details to manage. Check other posts on this website for information on how to graph rational functions. In this post, I look at one small clue that can help you figure out the behavior of a rational function as it approaches the vertical asymptotes. All you need to do is check the multiplicity of the factor in the denominator.

If the multiplicity of the factor is even, then the graph approaches +∞ from both sides of the asymptote, or it approaches -∞ from both sides of the asymptote.

If the multiplicity of the factor is odd, then the graph approaches +∞ on one side of the asymptote and approaches -∞ on the other side.

Here is an example that demonstrates this property:

\text{Graph } \dfrac {(x-2)(x+1)}{(x-1)(x+2)^2}

There are two vertical asymptotes for this function, at x=-2 and at x=1. The (x+2) factor is multiplicity 2 (even), so the graph approaches the same limit from both sides of the asymptote. The (x-1) factor is multiplicity 1 (odd), so the graph approaches opposite limits on either side of the asymptote. Here is the graph of the function, demonstrating this property:

Mar 16

Using multiplicity of factors to characterize graphs of polynomials

By Tutor GuyNo Comments

 

When you are asked to sketch the graph of a polynomial, you do not want to make a tree to calculate the values of various points. You don’t know where the “turning points” are, so you won’t be able to connect the dots for the points you plot. Instead, you need to fully factor the polynomial and use the zeroes you find to draw the polynomial. In addition, the multiplicity of each factor tells you whether the polynomial crosses the x-axis at that zero or “bounces”. The rule is very simple: If the factor has an odd multiplicity, the graph crosses the x-axis. If the multiplicity is even, the graph bounces.

multiplicity behavior at x ‑axis
odd crosses
even bounces

 

Example: Sketch the graph of

f(x)=x^3(x+1)(x-1)^2

Solution: First of all, plot the zeroes. For this problem, the zeroes are at x=-1, x=0, \text{ and } x=1.

 

 

 

 

 

 

 

Next, determine the degree of the polynomial. In this case, it is degree 6. (Add the exponents of all the factors: 3+1+2=6.) The degree tells you the end behavior, and you can draw arrows to show that the function will go to positive infinity on the left and the right.

 

 

 

 

 

 

 

 

Now you can sketch the graph. At x=-1, the zero is multiplicity 1, so the graph crosses the x-axis. At x=0, the zero is multiplicity 3, so the graph also crosses the x-axis. Note that for multiplicity 3, the graph doesn’t cross straight through the axis, but flattens out as it goes through. At x=1, the zero is multiplicity 2, so the graph bounces at the x-axis. The final sketch is shown below:

Feb 23

u substitutions with definite integrals

By Tutor GuyNo Comments

 

When a definite integral requires a u substitution to solve, be sure to substitute for the limits of integration as well. This way, you don’t need to substitute back in for the original function. Instead, you evaluate the integral using the new (u) limits. Here’s an example to show how this works.

Evaluate:

\displaystyle \int_0^{\pi/4} \sec^2 \theta \tan^2 \theta \; d \theta

This is an obvious candidate for a u substitution. (See other posts on this website for more information on when to use u substitutions.)

Let u= \tan \theta. Then du=\sec^2 \theta \; d \theta.

But don’t stop there! Use your expression for u to determine the new limits as well.

\theta =0 \rightarrow u=0; \; \theta= \dfrac{\pi}{4} \rightarrow u=1

So the new integral becomes

\displaystyle \int_0^{\pi/4} \sec^2 \theta \tan^2 \theta \; d \theta =\int_0^1 u^2 \; du= \left. \dfrac{1}{3}u^3 \right |_0^1=\dfrac{1}{3}-0=\dfrac{1}{3}

You have found the solution to the original integral without needing to put the integral back in terms of \theta.

Feb 16

Factoring ax² + bx + c, part 2

By Tutor GuyNo Comments

 

In my last post, I showed you a procedure for how to factor a quadratic when the leading coefficient is not equal to 1. Here I refine that method slightly with a short cut that makes your work even quicker. However, this method is a bit more difficult—if you are not really good at mental calculations, you will need to practice this a few times to get it down. To compare methods, I’ll use the same quadratic we factored in the last post.

Problem: Factor 12x^2+4x-5

  1. Our first step is the same as in the previous method—we need to find two numbers that multiply together to give 60 (that’s 12*-5) and add together to give +4. Of course, those numbers are the same as they were in the last post: +10 and -6.
    ……
  2. Now here’s the shortcut. Divide both of these numbers by the leading coefficient, 12. That gives two fractions:
    …..
    ….. \dfrac{10}{12} \;\; \text{and } \dfrac{-6}{12}
    …..
  3. Next, simplify the fractions if possible. In our example, we get
    …..
    ….. \dfrac{5}{6} \;\; \text{and } \dfrac{-1}{2}
    …..
  4. In each fraction, the denominator is the coefficient of the x term in our factor and the numerator is the constant term. So our two factors must be 6x+5 and 2x-1. See the diagram below:

 

 

Feb 09

Factoring ax² + bx + c

By Tutor GuyNo Comments

 

You probably have a lot of experience factoring quadratics of the form x^2+bx+c. (That is, where a, the coefficient on the x^2 term is equal to 1.) But what do you do when a isn’t 1? For example, let’s factor 12x^2+4x-5. You may have been taught a trial-and-error technique where you look for all the numbers that multiply to give 12x^2 and combine them with all the numbers that multiply to give 5 and test each one to see which gives the correct middle term. But there’s a simple algorithm that takes all the guesswork out. I’ll show you the procedure with an example.

Problem: Factor 12x^2+4x-5

  1. Similar to the case where a=1, look for two numbers that multiply together to give a \cdot c (in our example, 12 \cdot -5=-60) and add together to give b (i.e., +4 in our example). [Actually, you can see that this is just like the a=1 case, because there you were looking for two numbers that multiply together to give c, but when a=1, a \cdot c=c…] For our example, those numbers are +10 and -6 \\.
    …..
  2. Bring down the first term without changing it:
    …..
    ….. 12x^2
    …..
  3. Write the linear term as a sum or difference using the two numbers you found in step A.:
    …..
    ….. 12x^2 +10x-6x
    …..
    (Don’t worry about which term comes first. As it turns out, you can write these two terms in either order.)
    …..
  4. Bring down the last term without changing it:
    …..
    …..12x^2 +10x-6x - \; 5
    …..
  5. Now comes the magic. Look at the first two terms only from step D (cover up the last two terms if that helps you) and factor them:
    …..
    ….. 2x \!\!(6x+5)
    …..
  6. Now look at the last two terms in step D (again, cover the first two terms if that helps you) and factor them. And this is very important: if the x term has a minus sign on it, always factor out the minus sign:
    …..
    ….. 2x \!\! (6x+5) - \; 1 \!\! (6x+5)
    …..
    (Note that if nothing factors out of the last two terms, write a 1 (you can always factor 1 out of any term).
    …..
  7. Are the two expressions in the parentheses the same? They have to be. If they’re not, you’ve done something wrong. That’s one of the factors in your answer. The other factor is made up of the numbers outside the parentheses:
    …..
    ….. (2x-1) \!\! (6x+5)

 

Tada! I’ll leave it to you to show that if you had written the linear terms in step C in the reverse order, you’d get the same answer.

 

Feb 02

The addition rule and multiplication rule in probability

By Tutor GuyNo Comments

 

Two of the many formulas you learn in statistics are referred to as the Multiplication Rule and the Addition Rule. How do you figure out when to use each one? This is actually a pretty easy one. The multiplication rule is used to determine the probability of two events both happening, one after the other. The key word to look for in a problem is ‘and’.

Example 1: You draw a single card from a standard deck, replace it and draw a second card. What is the probability you draw a seven first AND a heart second? Because you want the probability of both events occurring, you use the multiplication rule.

The addition rule is used to find the probability that either one of two events occurs. The key word here is “or’.

Example 2: You draw a single card from a standard deck, replace it and draw a second card. What is the probability you draw a seven on the first draw OR a heart on the second draw? Because you want the probability of either event occurring, you use the addition rule.

How do the rules work? The multiplication rule comes in two forms. If the two events are independent, that is, if the probability of the second event does not change when the first event occurs, then the formula is simple:

 P(A \cap B) = P(A) \cdot P(B)

If the probability of the second event depends on the first event occurring, the formula is modified slightly to show this:

 P(A \cap B) = P(A) \cdot P(B|A)

You can see from either formula why this is called the multiplication rule. The addition rule is just slightly more complicated.

 P(A \cup B)=P(A)+P(B)-P(A \cap B)

You can see from this formula why it is called the addition rule. Let’s solve both problems.

Example 1: The two events are independent, so we use the first version of the rule:

 P(A \cap B) = P(A) \cdot P(B)=\dfrac{4}{52} \cdot \dfrac{13}{52}= \dfrac{1}{52}

Example 2: (Note that we use the result from Example 1 in solving this problem.)

 P(A \cup B)=P(A)+P(B)-P(A \cap B)=\dfrac{4}{52}+ \dfrac{13}{52}- \dfrac{1}{52}= \dfrac{16}{52}= \dfrac{4}{13}

Jan 26

Finding the inverse of a 3X3 matrix

By Tutor GuyNo Comments

 

What’s the easiest way to find the inverse of a 3×3 matrix? Use your graphing calculator, of course! But if you need to find the inverse without a calculator, here’s a method that will give you the solution with the least amount of trouble. We’ll demonstrate with an example.

Find the inverse of the following matrix:

 \begin{bmatrix} 1 & 4 & 7 \\ 2 & 5 & 8 \\ 3 & 6 & 10 \end{bmatrix}

The first step in finding the inverse is to calculate the determinant of the matrix. The easiest way to calculate a 3×3 determinant is to write the matrix out, and append the first two columns at the end:

\begin{bmatrix} 1 & 4 & 7 \\ 2 & 5 & 8 \\ 3 & 6 & 10 \end{bmatrix} \begin{matrix} 1 & 4 \\ 2 & 5 \\ 3 & 6\\ \end{matrix}

From here, you need to find the six different products along each of the diagonals shown:

 

 

 

 

 

 

Add the blue products together and add the red products together, then subtract the red total from the blue total: (50 + 96 + 84) - (105 + 48 + 80) = -3. This is the determinant. By the way, if the determinant is 0, stop. Your matrix does not have an inverse.

Next, you need to find the elements of the inverse matrix. Here’s a clever trick that will help you do that. Start by writing the transpose of the original matrix. This is done by changing all the rows into columns:

\begin{bmatrix} 1 & 2 & 3 \\ 4 & 5 & 6 \\ 7 & 8 & 10 \end{bmatrix}

Then write the first two columns over on the right and the first two rows over again on the bottom. Your array should look like this:

\begin{bmatrix} 1 & 2 & 3 \\ 4 & 5 & 6 \\ 7 & 8 & 10 \end{bmatrix} \begin{matrix} 1 & 2 \\ 4 & 5 \\ 7 & 8 \end{matrix} \\ \begin{matrix} \text{ }1 & 2 & 3 & \,\, 1 & 2 \\ \text{ }4 & 5 & 6 & \,\, 4 & 5 \end{matrix}

The next step is a little tricky to explain, though once you’ve done it, it’s pretty easy to figure out. For each of the nine positions in the matrix, you find the value of the determinant of the 2×2 array that is just below it and to the right. [One way to think of this is that each position in the matrix is the upper-left number of a 3×3 array. If you mentally delete the first row and column of that array, you have a 2×2 array left. This is the array for which you find a determinant. I demonstrate this below with the 1 and the 6 from the array above.


 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Do this process for all nine elements of the matrix and you should end up with this matrix:

\begin{bmatrix} 2 & 2 & -3 \\ 4 & -11 & 6 \\ -3 & 6 & -3 \end{bmatrix}

Finally, divide this matrix by the determinant you found above. The result will be the inverse of the original matrix:

\dfrac{1}{-3} \begin{bmatrix} 2 & 2 & -3 \\ 4 & -11 & 6 \\ -3 & 6 & -3 \end{bmatrix}= \begin{bmatrix} ^{-2} \! /_3 & ^{-2} \! /_3 & 1 \\ ^{-4} \! /_3 & ^{11} \! /_3 & -2 \\ 1 & -2 & 1 \end{bmatrix}

I’ll leave it to you to verify that this is indeed the inverse of the original matrix. For any 3×3 matrix that has an inverse, this method will calculate it for you correctly every time.

Jan 19

Completing the square

By Tutor GuyNo Comments

 

Are you an expert at completing the square? This is an important skill that is not nearly as difficult as a lot of students think it is. If you master just a couple of rules, you will be able to complete squares like a pro.

Let’s start with an explanation. Since we complete the square in algebra and not in geometry, exactly where is the square we are completing? This confuses many students and is probably the reason why they are frightened unnecessarily by the process. But it’s actually pretty simple. Let’s say we have the following expression:

(x-3)^2

This is obviously the square of (x-3), and so we call it a perfect square. If we expand the expression into x^2-6x+9, we recognize that it is still a perfect square. Any trinomial of the form ax^2+bx+c is a perfect square if it is the square of a binomial expression.

When we have an expression such as x^2-6x, we look for a number we can add to make it a perfect square of a binomial. In this case, adding 9 to x^2-6x to get x^2-6x+9 gives us the square of x-3. Therefore we have completed the square. That’s all the expression means!

Completing the square is actually quite easy in most cases. Because (x+a)^2=x^2+2ax+a^2, anytime we have an expression of the form x^2+2ax, all we need to do is add a^2. You can do this in two quick steps:

  1. Take the coefficient on the x term and divide it by 2.
  2. Square this number and add it to the end.

For example:

  • x^2+8x: Divide the 8 by 2 (you get 4) and square it (you get 16), so the completed square is x^2+8x \; + 16
  • x^2-12x: Divide the -12 by 2 (you get -6) and square it (you get 36), so the completed square is x^2-12x \; + 36
  • x^2+9x: Divide the 9 by 2 (you get \frac{9}{2}) and square it (you get \frac{81}{4}), so the completed square is x^2+9x \; + \frac{81}{4}
  • x^2-13x: Divide the -13 by 2 (you get \frac{-13}{2}) and square it (you get \frac{169}{4}), so the completed square is x^2-13x \; + \frac{169}{4}

Next you write the factor as a square. The factor is always of the form (x+a)^2 or (x-a)^2 where the value of a is the number that you squared. For the four examples above:

  • x^2+8x \; + 16 = (x + \; 4 )^2
  • x^2-12x \; + 36 = (x - \; 6 )^2
  • x^2+9x \; + \frac{81}{4} = (x + \; \frac{9}{2} )^2
  • x^2-13x \; + \frac{169}{4} = (x - \; \frac{13}{2} )^2

What if your expression starts with ax^2 where a is not equal to 1? Then you have to factor the a out of both terms before you complete the steps above. It can appear very complicated, but the process is the same every time. Here are some examples:

  • 2x^2+8x=2(x^2+4x) \rightarrow 2(x^2+4x+4)=2(x+2)^2
  • 4x^2-12x=4(x^2-3x) \rightarrow 4(x^2-3x+ \frac{9}{4}) = 4(x- \frac{3}{2})^2
  • 2x^2+9x=2(x^2+ \frac{9}{2}x) \rightarrow 2(x^2+ \frac{9}{2}x+ \frac{81}{16}) = 2(x+ \frac{9}{4})^2
  • 3x^2-13x=3(x^2- \frac{13}{3}x) \rightarrow 3(x^2- \frac{13}{3}x+ \frac{169}{36})=3(x- \frac{13}{6})^2
< Older
Blue Taste Theme created by Jabox