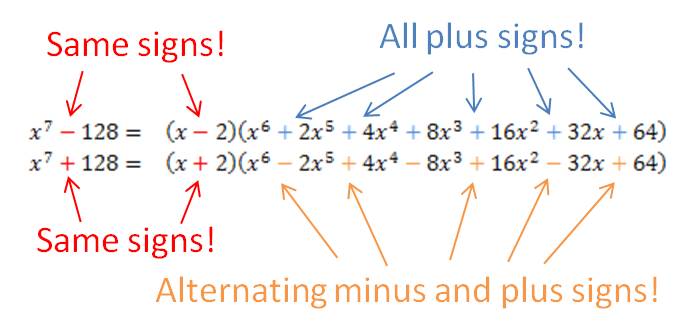
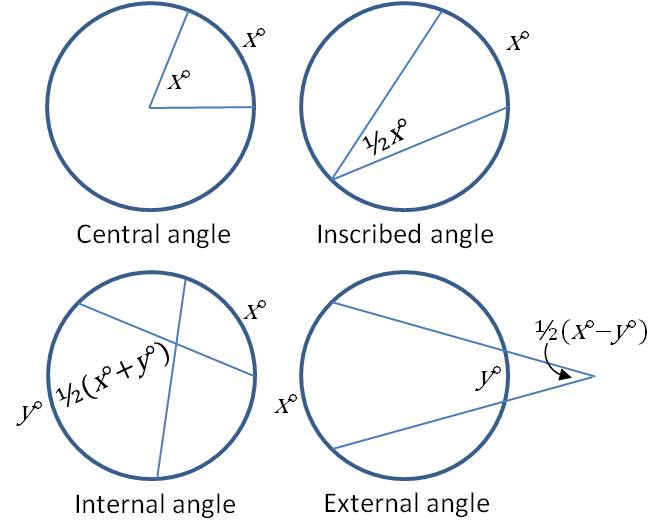
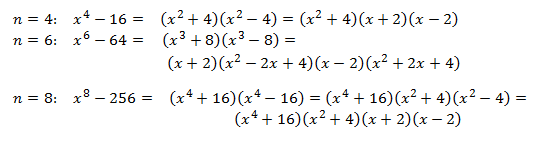Bay Area Tutoring

Strategies for finding integrals

By Tutor GuyNo Comments

How does one become an “expert integrator”? There are a lot of integration techniques, and recognizing which technique is the best is the first step to finding the integral. Many new calculus students look at an integral and don’t even know where to start. Here is a strategy you can adopt when faced with an integral. Carry on this internal dialog with yourself:

Is this something that I can integrate by inspection?
Is this an integral that can be turned into an elementary integral by simplifying?
Is there a trig identity that will simplify the integral?
Is it a candidate for a u substitution?
Can I use integration by parts?
Will decomposition by partial fractions be useful?
Does the integral involve a product of trig functions?
Would a trig substitution be effective?
Can I find it in a table of integrals?

Yes, this is a long list. But with practice, you can work your way through it pretty quickly. [If you are taking Calc AB this year, you will only learn the first four or five steps.] Think of each integration technique as a tool for your toolbox; with each integral you encounter, you decide which tool is the best for that problem.

Let’s look at a couple of examples to see how this works.

Example 1. Evaluate:

$\displaystyle \int {\sec}^2 x+x^2-2x \; dx$

This is an integral consisting of only elementary derivatives, so you can integrate it by inspection:

$\displaystyle \int {\sec}^2 x+x^2-2x \; dx = \tan x+ \dfrac{1}{3}x^3-x^2+C$

Note that you need to know the derivatives of the elementary functions in order to recognize when you can integrate by inspection. This means the derivatives of the trig functions, exponential and log functions and the inverse trig functions, not just polynomials.

Example 2. Evaluate:

$\displaystyle \int (x^2-1)(2x^2+3x) \; dx$

This is not an elementary derivative, so it cannot be integrated by inspection. But the integrand can by simplified by multiplying the terms out, turning it into an elementary derivative:

$\displaystyle \int (x^2-1)(2x^2+3x) \; dx \displaystyle \int 2x^4+3x^3-2x^2-3x \; dx=\dfrac{2}{5}x^5+\dfrac{3}{4}x^4-\dfrac{2}{3}x^3- \dfrac{3}{2}x^2+C$

Example 3. Evaluate:

$\displaystyle \int \tan^2 x \; dx$

This is not an elementary derivative. Is there a trig identity that will help? Yes, use the following Pythagorean identity to turn this into a simple integral:

$\displaystyle \int \tan^2 x \; dx = \displaystyle \int \sec^2 x-1 \; dx = \tan x-x+C$

At this point, you might be thinking “how am I supposed to see that identity?” Well, there’s no substitute for experience and practice. It’s important to know your basic trig identities, so you can rewrite a trig integral in a different form. If one identity doesn’t work, try another. Finding integrals is as much an art as it is a science. Be creative and be inventive.

(Look for other tips on integral problems on this website for strategies on u substitution, integration by parts, products of trig functions and trig substitutions.)

Dec 29

Sum( and Seq( commands on your calculator

By Tutor GuyNo Comments

Can you find the sum of the following series?

$\displaystyle \sum_{i=1}^{15}\dfrac{2n+1}{3n-2}$

This is neither an arithmetic nor a geometric series, so you don’t have a formula for it. This would be a tedious problem to do by hand. Fortunately, your graphing calculator can do these problems quickly and efficiently.

There are two functions you need to use on your calculator. The seq( command creates a sequence of terms based on a rule that you give. The sum( command adds together the terms in a sequence. Both functions are found on the LIST menu on your calculator. The seq( command is on the OPS submenu and the sum( command is on the MATH submenu.

To sum a series, you combine the two commands. If you have the new operating system on your calculator, it will prompt you for the entries when you select the seq( command. If you have the old operating system, you need to know the syntax for the command. The syntax for the series above is:

sum(seq((2x + 1)/(3x – 2),x,1,15))

Note that the seq( command has four parameters in the parentheses. From left to right, these are 1) the rule for the nth term of the sequence; 2) the variable name; 3) the first value of the variable; and 4) the final value of the variable. Now all you need to do is type this in to your calculator and let it do the crunching:

Dec 22

The “parent” functions

By Tutor GuyNo Comments

Do you know your parent functions? I’m surprised every year when some of my students don’t know how to graph some of the elementary functions they’ve learned in class. Your chances for success in precalc and calculus are significantly better if you memorize the shapes of these ten functions. No excuses, no shortcuts. Just learn them.

y = xⁿ (Power functions)

y = |x| (absolute value function)

y = ⌊x⌋ (Greatest integer function)

y = √x

y = 1/x

y= b^x (exponential function – growth and decay)

y = log x

y = sin x and y = cos x

y = tan x

Dec 15

Calculating probabilities as a fraction

By Tutor GuyNo Comments

The first day you are asked to find probabilities, the questions are relatively simple:

What is the probability of rolling a 2 or a 3 on a standard die? [Ans: 2/6 = 1/3]
What is the probability of drawing a five from a standard deck of cards? [Ans: 4/52 = 1/13]

But very quickly, the problems get a lot more complicated:

What is the probability of flipping a coin ten times and getting exactly 8 heads?
What is the probability of being dealt a full house (three of a kind plus a pair) in a five card poker hand?
A class has 14 boys and 12 girls. If the teacher randomly creates a new seating chart, what is the probability that the six students in the front row comprise exactly four boys and two girls?

How do you attack these problems? The best way is to think of probability as a fraction. The numerator counts all the ways the specified event can occur, and the denominator counts all the ways any event can occur:

Your event
Any event

Then you use combinatorics to count all the possibilities on the top and on the bottom. Let’s see how we can use this method to answer the three questions posed above:

$st-0401-frac$

(Note that this question could also be treated as a binomial probability and solved that way.)

$st-0402-frac$

Dec 08

Factoring sums and differences of fifth powers (and higher!) when the power is odd

By Tutor GuyNo Comments

Occasionally, you are required to factor a polynomial that is in the form of a sum or difference of two power terms (i.e., xⁿ– yⁿ). In my last post, I showed you a simple procedure you can learn to help you factor the binomial if the value of the power is 4 or greater and the power is even. [If you are reading this post, you should already be familiar with factoring the difference of two squares and the difference of two cubes. Check this website for posts on those procedures.] In this post, I show you a procedure (more complicated than the others, I admit) that you can use to factor these binomials when the power is odd.

First, let’s see how this works on some examples:

Do you see the pattern? When factoring aⁿ– bⁿ or aⁿ+ bⁿ (for n odd), there is always a linear term a ± b, where the plus or minus sign is the same as in the original binomial. The remaining factor is a little more complicated, but it does have a simple pattern. Each term consists of the first term in the binomial (with its exponent decreasing from n – 1 to 0) and the second term in the binomial with its exponent increasing from 0 to n – 1. In the first example above, the x term decreased from x⁴ to x⁰. The 2 term increased from 2⁰ to 2⁴. If you started with the difference of two powers, all of the signs in the second factor are plus signs. If you started with the sum of the two powers, the first sign in the second factor is a minus sign and the signs alternate after that.

Dec 01

Factoring differences of fourth powers (and higher!) when the power is even

By Tutor GuyNo Comments

Occasionally, you are required to factor a polynomial that is in the form of a difference of two power terms (i.e., xⁿ – yⁿ). There is a simple procedure you can learn to help you factor the polynomial if the value of the power is 4 or greater and the power is even. [If you are reading this post, you should already be familiar with factoring the difference of two squares and the difference of two cubes. Check this website for posts on those procedures.]

First, let’s see how this works on some examples:

You can see that whenever the power is even, you treat the binomial as the difference of two squares and find its factors. If the factors are still the sum and difference of perfect squares, you repeat the process with the term that is the difference of two squares. If the factors are now the sum and difference of perfect cubes, you follow the method for factoring the sum or difference of two cubes. If the powers are sums and differences of odd powers higher than 3, there is another procedure to factor them. You can find another post on this website that shows that procedure.

Nov 10

Divisibility rules

By Tutor GuyNo Comments

You always need to simplify fractions and radicals before you box your final answer. In order to simplify these, you need to be able to factor integers. There was a teacher at my middle school who urged his students to “learn your gozintas!” In other words, know whether a number goes into the number you are factoring. Now that calculators are so common, many students today don’t think they need to learn their gozintas. However, the divisibility rules for the first six prime numbers are so easy to learn, you will benefit from knowing them.

A number is divisible by ___ if:		Examples
2	Last digit is 0, 2, 4, 6, 8	478 ends in 8, so it is divisible by 2. 8349 doesn’t end in 0, 2, 4, 6 or 8 so it is not divisible by 2.
3	Sum of digits is divisible by 3	8349 is divisible by 3 because 8+3+4+9 = 24 which is divisible by 3. [See note below.] 1450 is not divisible by 3 because 1+4+5+0 = 10 which is not divisible by 3.
5	Last digit is 0, 5	1450 is divisible by 5. 3157 is not divisible by 5.
7	Twice the last digit subtracted from the remaining digits is divisible by 7 (Take the last digit and double it. Subtract this from the original number after the last digit is removed.)	3157 is divisible by 7 because 315 – 27 = 315-14 = 301 which is divisible by 7. [See note below.] 4563 is not divisible by 7 because 456 – 23 = 456 – 6 = 450 which is not divisible by 7.
9	Sum of digits is divisible by 9	4563 is divisible by 9 because 4+5+6+3 = 18 which is divisible by 9. [See note below.] 90827 is not divisible by 9 because 9+0+8+2+7 = 26 which is not divisible by 9.
11	Sum of odd digits – sum of even digits is divisible by 11 (Here, odd and even digits refer to their place in the number, not their value. For example, if you have a 9 digit number, add the 1^st, 3^rd, 5^th, 7^th and 9^th digits and subtract the sum of the 2^nd, 4^th, 6^th and 8^th digits. If this number is divisible by 11, the original number is divisible by 11.)	90827 is divisible by 11 because (9+8+7) – (0+2) = 24 – 2 = 22 which is divisible by 11. [See note below.] 478 is not divisible by 11 because (4+8)-7 = 5 which is not divisible by 11.

Note: What if you do the test for divisibility by 3, 7, 9 or 11, but you don’t know whether the number you obtained is divisible by the number you are testing? Then just do the test again. Let’s test a large number to see how this works.

Example 1: Is 999,999 divisible by 3 or by 9?

9+9+9+9+9+9 = 54. And 5+4 = 9. So 999,999 is divisible by 3 and by 9.

Example 2: Is 999,999 divisible by 7?

99,999 – 18 = 99,981. And 9998 – 2 = 9986. And 998 – 12 = 986. And 98 – 12 = 84. And 8 – 8 = 0, which is divisible by 7. So 99,999 is divisible by 7! (And so are 99,981 and 9986 and 986 and 84!)

No matter how large your original number, you can just keep repeating the test until you know your answer for certain.

Nov 03

Surface area and volume formulas for solids

By Tutor GuyNo Comments

Solid figures can be scary for a lot of students. There are a lot of confusing formulas and it can be difficult to draw a three-dimensional figure on a flat sheet of paper. But solids can be tamed if you categorize them by the number of bases. Note in the table below that the formulas for prisms and cylinders are exactly the same, as are the formulas for pyramids and cones. You will have the most success if you memorize these formulas—then you just plug in the given values to find the unknown values. If you are struggling with the formulas, print this page out and paste it into your binder until you are more comfortable with them.

Bases	Figure	Lateral surface area	Volume
2	Prism	$ph$	$Bh$
2	Cylinder	$ph = 2 \pi rh$	$Bh= \pi r^2h$
1	Pyramid	$1/2 p \ell$	$1/3 Bh$
1	Cone	$1/2 p \ell = \pi r \ell$	$1/3 Bh = 1/3 \pi r^2h$
0	Sphere	$4 \pi r^2$	$4/3 \pi r^3$

p = perimeter; h = height; ℓ = slant height; B = Base area; r = radius

An added wrinkle that can make pyramids difficult is that you are not always given all the necessary information to plug into the formulas. What do you do? Remember what you’ve learned about regular polygons and use the Pythagorean Theorem! Let’s look at an example. The pyramid in the figure is a regular pyramid with a hexagonal base. The edge length is 6 cm and the radius of the base is 3 cm. Find the lateral surface area and the volume of the pyramid.

You can see from the pyramid formulas above that we need to know the perimeter of the base and the slant height to find the area, and we need to know the Base area and the height to find the volume. And we don’t have any of these numbers! It seems like we don’t have enough information. But there was one other clue that will help us solve this puzzle. The pyramid is regular, which means the foot of the altitude touches the center of the base, and the base is regular. If you draw the altitude, it forms a right triangle with the radius and the edge.

Using the Pythagorean Theorem (or recognizing this as a 30°-60°-90° triangle), you can easily find the altitude is

$h=3 \sqrt{3}$

Because the base is a regular hexagon, you can also find the edge length, perimeter and the apothem:

$s=3\rightarrow p=18; a= \dfrac{3 \sqrt{3}}{2}$

Finally, the triangle formed by the altitude, apothem and slant height is also a right triangle.

From the Pythagorean Theorem again, we find the slant height:

${\ell}^2={\left( \dfrac{3 \sqrt{3}}{2} \right)}^2 +{\left( 3 \sqrt{3} \right)}^2= \dfrac{135}{4} \rightarrow \ell = \dfrac{3 \sqrt{15}}{2}$

Now we have all the information we need to find the area and volume. Just plug it all in:

$LA = \dfrac{1}{2} p \ell = \dfrac{1}{2}(18)\!\!\left( \dfrac{3 \sqrt{15}}{2} \right) = \dfrac{27 \sqrt{15}}{2}$

$V = \dfrac{1}{3}Bh=\dfrac{1}{3} \! \left( \dfrac{1}{2}ap \right)\! h= \dfrac{1}{3} \cdot \dfrac{1}{2} \!\left( \dfrac{3 \sqrt{3}}{2} \right) \! (18)(3 \sqrt{3})= \dfrac{81}{2}$

Whew! Yes, that’s a lot of work, but just break the problem down piece by piece and you can solve these problems like a pro!

Oct 27

Simplifying “3-stack” and “4-stack” fractions

By Tutor GuyNo Comments

I had a physics student a number of years ago who worked a complicated problem and ended up with the following (I’ve changed the actual numbers to make our work here easier to follow):

$\dfrac{(2)(3)}{4}=\dfrac{(5)(6)(7)B}{(8)(9)}$

Of course, his next step was to solve for B. It should be clear to you, as it was to him, that he needed to multiply both sides by 8 and 9 and divide by 5, 6 and 7. That is what he did. But this is how he wrote the solution:

$B= \dfrac{9 \cdot \left( \dfrac{ \left(\dfrac{ 8 \cdot \left( \dfrac{(2)(3)}{4} \right)}{5} \right)}{6} \right)}{7}$

His expression was totally correct, and he found the correct value of B, but he made the problem so much harder than he needed to. He created a fraction with five different stacks in it and he needed all those parentheses to keep track of which number was a numerator and which was a denominator.

This is an extreme case of what I see so many students do: they create “3-stack” and “4-stack” fractions all the time when they are simplifying problems. Let’s look at two examples. I’ll solve them with “bad” solutions and then with much smarter solutions (I don’t simplify the answers here because I want to focus on the first step of the solution):

Example 1. Solve:

$3x= \dfrac{2}{5}$

Bad solution:

$x= \dfrac{\dfrac{2}{5}}{3}$

Smarter solution:

$x= \dfrac{2}{5 \cdot 3}$

Example 2. Solve:

$\dfrac{4}{7}x=\dfrac{2}{5}$

Bad solution:

$x= \dfrac{\dfrac{2}{5}}{\dfrac{4}{7}}$

Smarter solution:

$x= \dfrac{2 \cdot 7}{5 \cdot 4}$

You can see that the smarter solutions are simpler to read and easier to simplify.

It is easy to train yourself to write answers as “2-stack” fractions if you remember one simple rule:

Respect the vinculum.

Um, respect the what?!?

The vinculum. When you write a fraction, the horizontal line that separates the numerator from the denominator is called the vinculum. No one ever talks about it, but it’s a very powerful symbol. It tells you to multiply by every number that is above it and divide by every number that is below it. And it’s as easy as that. So when you are simplifying expressions like the ones in the two examples above or the more complicated example at the beginning of this post, all you need to do is to put numbers that are multiplied above the vinculum and numbers that are divided below the vinculum. And what happens if you are multiplying or diving by a fraction? Then put the numerator on top and the denominator on the bottom if you are multiplying. Flip the fraction over first if you’re dividing (as I did in Example 2). When you do this, you will end up with a 2-stack fraction that can be easily evaluated. Let’s look at the original problem again:

$\dfrac{(2)(3)}{4}=\dfrac{(5)(6)(7)B}{(8)(9)}$

To solve for B, you will multiply by 8 and 9, so they go on top. And you will divide by 5, 6 and 7, so they go on the bottom. In one step, you’ve solved for B as follows:

$B= \dfrac{2 \cdot 3 \cdot 8 \cdot 9}{5 \cdot 6 \cdot 7}$

Compare that to the monstrosity at the beginning. So much easier!

P.S. You don’t really have to remember the name “vinculum”. Most people don’t know what the line is called and don’t care. Chances are good your math teacher doesn’t even know the term. To make things even more bizarre, when you write a fraction with a slash instead of a horizontal line like this—2/3 – the slash is called a “virgule”. Most people don’t know that one and don’t care about it either. All you have to remember is that the horizontal line in a fraction tells you to multiply on top and divide on the bottom.

Oct 20

Angles in circles and arc measures

By Tutor GuyNo Comments

When angles are formed by chords, secants and tangents in a circle, there are several formulas that help you determine the arc measures and angle measures that are formed. With a little bit of thought, you can often find values for all of the arcs and angles around the circle. For many students, the equations are difficult to remember. Your best approach is to categorize the angle by the location of the vertex. Then learn the rule for the angles with that particular vertex. There are four possibilities:

Central angles: This is an angle whose vertex is at the center of the circle. For all central angles, the angle measure is equal to the arc measure of the subtended arc.
Inscribed angles: These angles have their vertex on the circle. They can be chord-chord angles or chord-tangent angles. For these angles, the angle measure is one half the arc measure of the subtended arc.
Internal angles: These are angles whose vertex is in the interior of the circle but not at the center. For these angles, the angle measure is one half the sum of the arc measures of the two subtended arcs.
External angles: These are angles whose vertex is outside the circle. They can be secant-secant angles or secant-tangent angles. For these angles, the angle measure is one half the difference of the arc measures of the subtended arcs.

Archive

The blog archives.

Strategies for finding integrals

Sum( and Seq( commands on your calculator

The “parent” functions

Calculating probabilities as a fraction

Factoring sums and differences of fifth powers (and higher!) when the power is odd

Factoring differences of fourth powers (and higher!) when the power is even

Divisibility rules

Surface area and volume formulas for solids

Simplifying “3-stack” and “4-stack” fractions

Angles in circles and arc measures

Tutor Guy Homework Tips